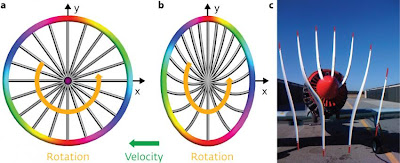
Here's why: "When an object is moving close to the speed of light, relativistic effects occur. For example, to an external observer an object moving very fast appears squeezed in the direction of the object’s motion (Figure above). This so-called Lorentz contraction arises from the timing in which light from the fast-moving object arrives at the observer. Bliokh and Nori have now shown that if such an object also rotates at the same time, for example a flywheel, then the rotating motion is also affected. The spokes in the wheel appear distorted in a way that makes them seem denser in one direction than the other."
On the other hand, a rolling shutter with carefully chosen curtain speed in the direction of movement can compensate for, at least, the squeezed shape of the wheel.
P.S. A Youtube video explains the propeller picture:
Very nice video illustration!
ReplyDeleteMaybe I would have reversed the shading gradient at the shutter position since the integration starts off dark and then brightens a few rows later, but then again, I don't think the illustration would have been as clear.
Par google traudction ??
ReplyDeleteA poor analogy I think. Relativistic effects depend on the direction of the particle/wave's travel. Rolling shutter effects depends on both the object's travel and the direction of sensor readout.
ReplyDeleteWith rolling shutter objects traveling in one direction get compressed. Travel in the other direction and objects get stretched. Any other direction and they get sheared and bent. Let's see relativity do that.
A square is like a cube, but not really.
May be poor. I meant that we used to think that global shutter always produces undistorted image, while rolling shutter distorts moving objects. However, at relativistic speeds the global shutter always distorts objects, while a carefully chosen rolling shutter travelling in certain direction can produce undistrorted image. Obviously, the direction and speed to the rolling shutter can not be arbitrary to get this compensation. But the global shutter can not compensate for relativistic compression at all.
DeleteI think it's an OK analogy, although I'm not claiming it's a mathematically exact one. The reason this analogy is fair is that the important quantities in the rolling shutter (speed of rotation, velocity of shutter) carry over to the relativistic problem of a rotating wheel whose center is moving with some velocity (speed of rotation, relative velocity between wheel's center and observer). So both of these effects depend on two things, a rotational speed, and also some velocity which picks out an axis along which stretching occurs. So when you say "Let's see relativity do that.", it actually already does.
DeleteIn fact, the analogy holds more water than one might think. Suppose the wheel is translating to the right. If we ignore Lorentz contraction and just consider time dilation, Then the Lorentz Transformation really IS just a spacetime skew transformation. That is, events at various positions observed at the same time along the direction of velocity according to the observer actually occurred at different times in the frame moving with the wheel. In fact, this relationship is linear, so every meter moved along the direction of velocity corresponds to the same change in time between when observed events happened in the moving frame. In this approximation, the rolling shutter effect is more or less the same as a relativistic translating wheel, because positions along the direction of shutter velocity reveal events that actually happened at different times in the same linear way.
How often do you take pictures of objects moving close to the speed of light?
ReplyDelete